Mastering The 3 Variable System Of Equations: A Comprehensive Guide
Understanding the intricacies of a 3 variable system of equations is essential for students, engineers, and anyone who deals with mathematical modeling. This topic provides a foundation for various applications in science, technology, and economics. Solving these equations can be daunting, but with the right approach, it becomes an achievable task. By grasping the methods available, individuals can unlock a deeper understanding of the relationships between variables and their impact on real-world scenarios.
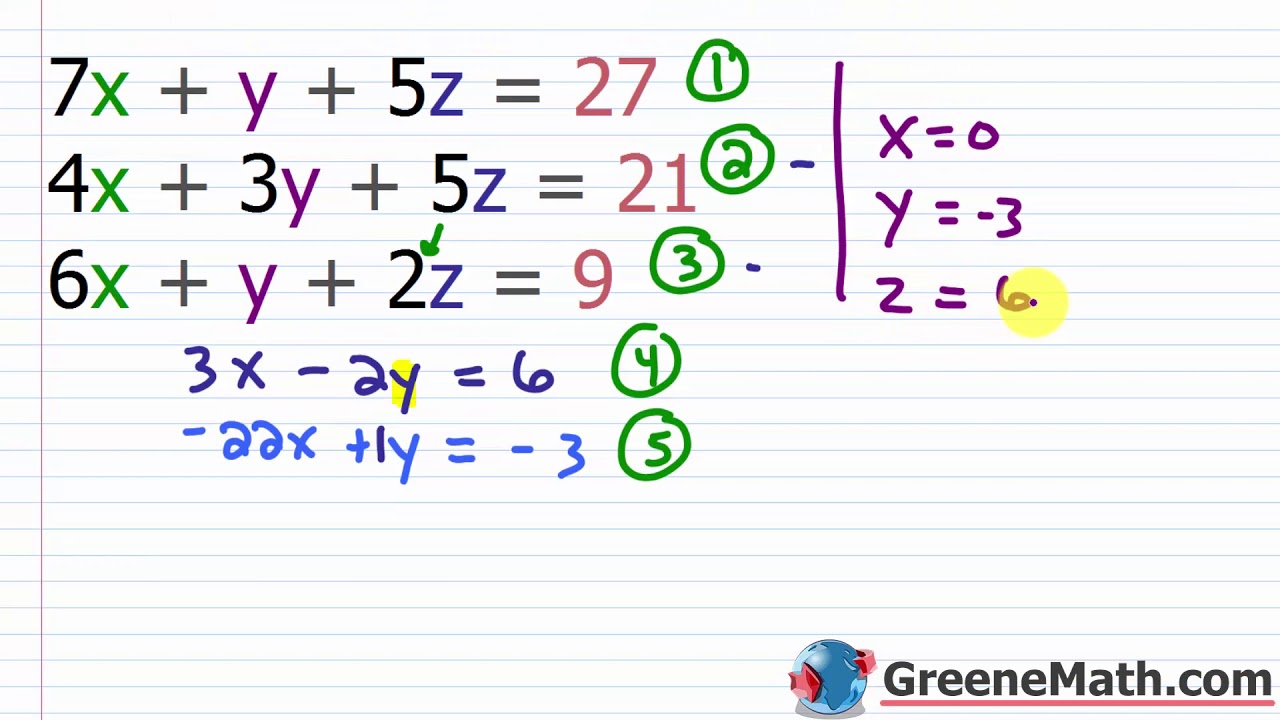
The 3 variable system of equations consists of three equations with three different variables, often expressed as x, y, and z. To find a solution, one must determine the values of these variables that satisfy all three equations simultaneously. This system can be solved using different techniques such as substitution, elimination, or matrix methods. Each method offers unique advantages and insights into the relationships between the variables involved.
In practical applications, the 3 variable system of equations can be utilized in various fields such as physics for analyzing forces, in economics for optimizing resource allocation, or in engineering for designing complex systems. As technology continues to evolve, the importance of mastering these equations becomes even more critical. This article aims to provide a thorough understanding of the 3 variable system of equations, its applications, and the methods used to solve it effectively.
What is a 3 Variable System of Equations?
A 3 variable system of equations consists of three linear equations that involve three different variables. The general form of such a system is:
- Equation 1: ax + by + cz = d
- Equation 2: ex + fy + gz = h
- Equation 3: ix + jy + kz = m
Where a, b, c, d, e, f, g, h, i, j, k, and m are constants. The solution to this system is the set of values (x, y, z) that satisfy all three equations simultaneously.
How Do You Solve a 3 Variable System of Equations?
There are several methods to solve a 3 variable system of equations, including:
1. Substitution Method
This method involves solving one equation for one variable and substituting that expression into the other equations. This process continues until all variables are determined.
2. Elimination Method
The elimination method involves manipulating the equations to eliminate one variable at a time, making it easier to solve for the remaining variables.
3. Matrix Method
This method uses matrices and determinants to find the solution. It is particularly useful for larger systems of equations or when dealing with complex relationships.
What Are the Applications of a 3 Variable System of Equations?
The 3 variable system of equations has numerous real-world applications, including:
- Physics: Analyzing forces acting on an object.
- Economics: Optimizing production levels in businesses.
- Engineering: Designing systems with multiple interacting components.
- Statistics: Modeling relationships between variables in data analysis.
Can All 3 Variable Systems Be Solved?
Not all 3 variable systems have a unique solution. The possible outcomes for a 3 variable system of equations include:
- A unique solution: The system has one set of values for (x, y, z).
- No solution: The equations represent parallel planes that do not intersect.
- Infinitely many solutions: The equations represent the same plane or coincide at a line.
How Do You Check the Solution of a 3 Variable System of Equations?
To verify that a solution is correct, substitute the values of (x, y, z) back into the original equations. If all three equations are satisfied, the solution is valid. Otherwise, it may require reevaluation or correction.
Example of a 3 Variable System of Equations
Consider the following system:
1. 2x + 3y + z = 1 2. x - y + 2z = 4 3. 3x + 2y - z = 2
By applying any of the mentioned methods, you can find the values of x, y, and z that satisfy all three equations.
What Resources Are Available for Learning About 3 Variable Systems?
There are numerous resources available for mastering the 3 variable system of equations, including:
- Online courses and tutorials.
- Textbooks on algebra and linear equations.
- Online forums and study groups.
- Educational YouTube channels focused on mathematics.
What Are Common Mistakes When Solving 3 Variable Systems?
Some common mistakes include:
- Making arithmetic errors during calculations.
- Incorrectly substituting values into the equations.
- Failing to check the solution against all original equations.
Conclusion
In conclusion, the 3 variable system of equations is a fundamental concept in mathematics with vast applications across various fields. By understanding how to solve these systems effectively, individuals can enhance their problem-solving skills and apply them to real-world scenarios. Whether through substitution, elimination, or matrix methods, mastering these techniques will provide a solid foundation for further study in mathematics and its applications.