Exploring The Concept Of Limits Infinity In Mathematics
In the world of mathematics, the concept of limits and infinity plays a crucial role in understanding functions and their behaviors as they approach certain values. As we delve into this fascinating topic, it becomes evident that limits are not merely abstract ideas but are foundational to calculus and mathematical analysis. The interplay between limits and infinity invites us to explore deeper philosophical questions about the nature of numbers and the infinite continuum they represent.
As we embark on this journey, we will unravel the intricacies of limits and infinity, shedding light on how these concepts intersect in various mathematical contexts. From the basic definition of limits to their applications in real-world scenarios, we will illustrate the importance of these ideas in both academic and practical settings. Understanding limits infinity is essential for students and professionals alike, as it opens doors to advanced topics in mathematics and related fields.
So, what does it mean when we talk about limits approaching infinity? What are the implications of this concept in calculus, and how can we visualize it? Join us as we explore these questions and more, bringing clarity to the sometimes perplexing world of limits and infinity.
What Are Limits in Mathematics?
Limits are fundamental concepts in calculus that describe the behavior of a function as its input approaches a certain value. A limit can be thought of as the value that a function approaches as the variable gets closer to a specific point. This concept is crucial for defining derivatives and integrals, which are cornerstones of calculus.
Why Is the Concept of Infinity Important in Limits?
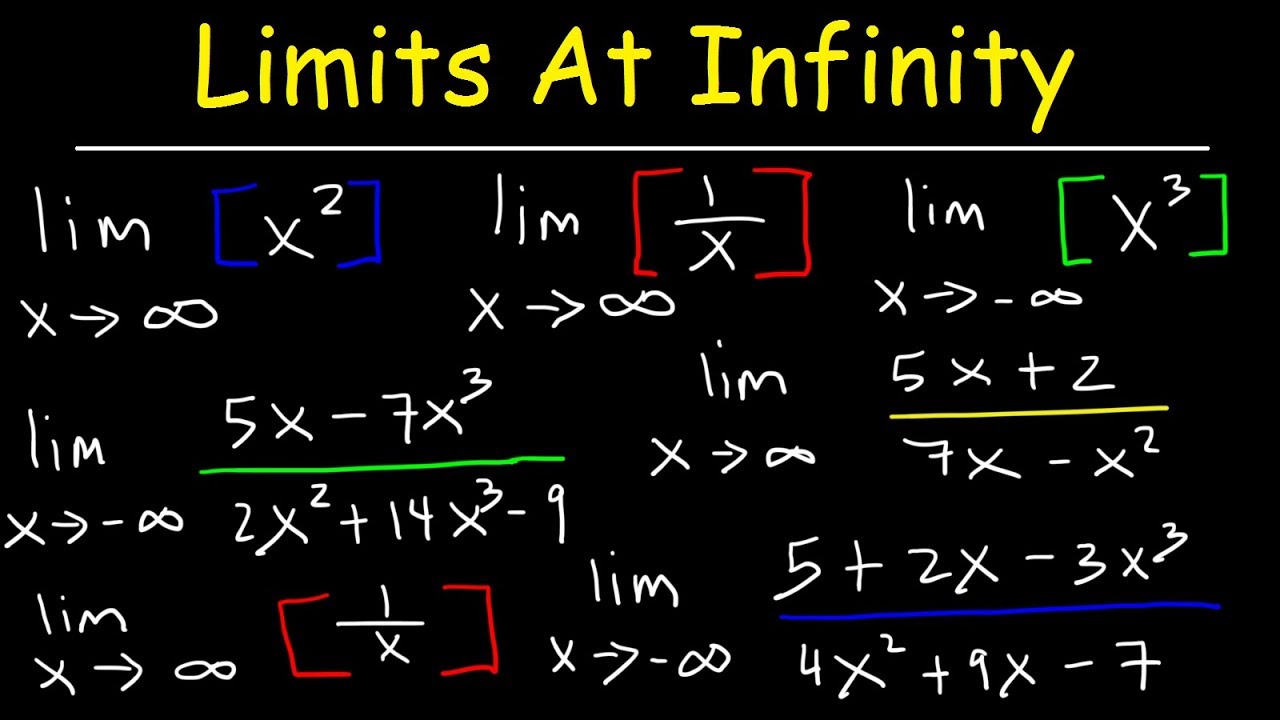
Infinity represents an unbounded quantity that is larger than any finite number. When discussing limits, we often encounter scenarios where the input variable approaches infinity. This leads to questions about how functions behave as they grow indefinitely. Understanding how limits behave at infinity allows mathematicians to analyze functions that might otherwise seem unpredictable.
How Do We Calculate Limits?
Calculating limits can be done through several methods, including:
- Direct Substitution
- Factoring
- Rationalizing
- Using L'Hôpital's Rule
Each of these methods has its own applications and is chosen based on the specific function being analyzed.
What Is the Relationship Between Limits and Infinity?
The relationship between limits and infinity is a pivotal aspect of calculus. When we discuss limits approaching infinity, we are often interested in the end behavior of functions. This means examining how a function behaves as it increases or decreases without bound. For instance, consider the limit of the function f(x) = 1/x as x approaches infinity. As x grows larger, f(x) approaches 0, which illustrates how limits can provide insights into function behavior at extreme values.
What Are One-Sided Limits?
One-sided limits refer to the behavior of a function as it approaches a specific point from one side only—either from the left (denoted as lim x→c-) or from the right (denoted as lim x→c+). This is particularly useful when dealing with functions that have discontinuities or undefined points. Understanding one-sided limits helps to clarify how a function behaves near these critical values.
How Do Limits Infinity Apply to Real-World Scenarios?
The concept of limits infinity has real-world applications in various fields, including physics, engineering, and economics. For example:
- In physics, limits are used to describe motion approaching terminal velocity.
- In economics, limits can model diminishing returns as resources become scarce.
- In engineering, limits can help calculate load capacities as structures approach their breaking point.
Can Limits Infinity Be Visualized?
Visualizing limits infinity is a powerful tool for understanding their implications. Graphs of functions can illustrate how values approach infinity or how they behave near critical points. By plotting functions, mathematicians and students can see firsthand how limits operate, providing a concrete representation of abstract concepts.
What Are Some Common Misconceptions About Limits Infinity?
There are several misconceptions surrounding limits and infinity, including:
- Confusing infinity with a number: Infinity is not a fixed value but an idea that represents unboundedness.
- Assuming limits always exist: Not all functions have limits at every point, especially at discontinuities.
- Believing that limits at infinity always equal zero: This is not true; functions can approach any finite value or even remain infinite.
What Is the Importance of Understanding Limits Infinity in Advanced Mathematics?
Understanding limits infinity is vital for anyone pursuing advanced studies in mathematics or related fields. It lays the groundwork for more complex topics such as:
- Calculus and Differential Equations
- Real Analysis
- Complex Analysis
A firm grasp of these concepts can lead to deeper insights and a more profound appreciation for the beauty of mathematics.
In conclusion, the exploration of limits infinity serves as a gateway to understanding some of the most profound and intriguing aspects of mathematics. From calculating limits to visualizing their behavior, the journey through limits infinity is not only educational but also enriching, revealing the elegance of mathematical thought.