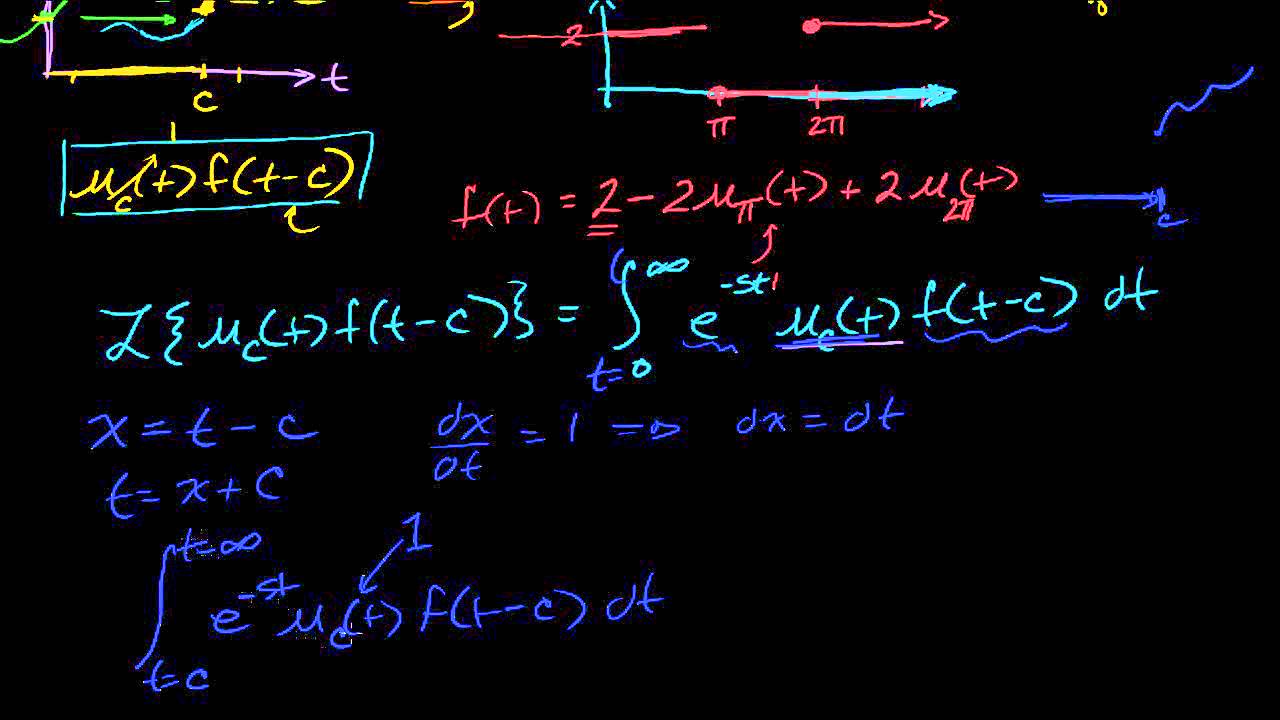Differential Equations And Laplace Transforms: A Comprehensive Guide

Differential equations and Laplace transforms are fundamental concepts in mathematics and engineering, playing a crucial role in various applications across science and technology. Understanding these concepts is essential for students, professionals, and researchers alike. In this article, we will explore the intricacies of differential equations, the utility of Laplace transforms, and how these two mathematical tools interact with each other to solve complex problems in various fields.

As we delve into this subject, we will provide a thorough analysis of different types of differential equations, the principles behind Laplace transforms, and their applications in real-world scenarios. This comprehensive guide aims to equip readers with the knowledge to tackle differential equations effectively and utilize Laplace transforms to simplify problem-solving processes.

Whether you are a student grappling with these concepts for the first time or a seasoned professional looking to refresh your knowledge, this article will serve as a valuable resource. By the end, you will have a deeper understanding of differential equations and Laplace transforms, empowering you to apply these tools confidently in your academic or professional endeavors.

Table of Contents

• Introduction to Differential Equations
• Types of Differential Equations
• Methods for Solving Differential Equations
• Introduction to Laplace Transforms
• Properties of Laplace Transforms
• Applications of Laplace Transforms
• Connection Between Differential Equations and Laplace Transforms
• Conclusion

Introduction to Differential Equations

Differential equations are mathematical equations that involve an unknown function and its derivatives. They describe how a quantity changes concerning another variable, typically time or space. In various fields, including physics, engineering, and economics, differential equations model dynamic systems and processes.

There are two main types of differential equations: ordinary differential equations (ODEs) and partial differential equations (PDEs). ODEs involve functions of a single variable, while PDEs involve functions of multiple variables. Understanding the distinction between these types is crucial for applying the appropriate solution methods.

Types of Differential Equations

Ordinary Differential Equations (ODEs)

Ordinary Differential Equations (ODEs) can be classified into several categories:

• Linear vs. Nonlinear ODEs: Linear ODEs can be expressed in a linear form, while nonlinear ODEs do not follow this structure.
• Homogeneous vs. Nonhomogeneous ODEs: Homogeneous ODEs have solutions that pass through the origin, while nonhomogeneous ODEs contain a constant term.
• First Order vs. Higher Order ODEs: First-order ODEs involve the first derivative, while higher-order ODEs involve derivatives of second order or higher.

Partial Differential Equations (PDEs)

Partial Differential Equations (PDEs) are more complex than ODEs and involve functions of multiple variables. They can also be classified into categories:

• Linear vs. Nonlinear PDEs: Similar to ODEs, PDEs can be either linear or nonlinear.
• Elliptic, Parabolic, and Hyperbolic PDEs: These classifications are based on the characteristics of the equations and the type of boundary conditions involved.

Methods for Solving Differential Equations

Solving differential equations can be challenging, but several methods can simplify the process:

• Separation of Variables: This method is effective for first-order ODEs, allowing the separation of variables to solve the equation.
• Integrating Factor: This technique is useful for linear first-order ODEs, where an integrating factor is applied to facilitate the solution.
• Characteristic Equation: This method is often used for higher-order linear ODEs to find particular solutions.
• Numerical Methods: When analytical solutions are difficult to obtain, numerical methods such as Euler's method or Runge-Kutta methods can provide approximate solutions.

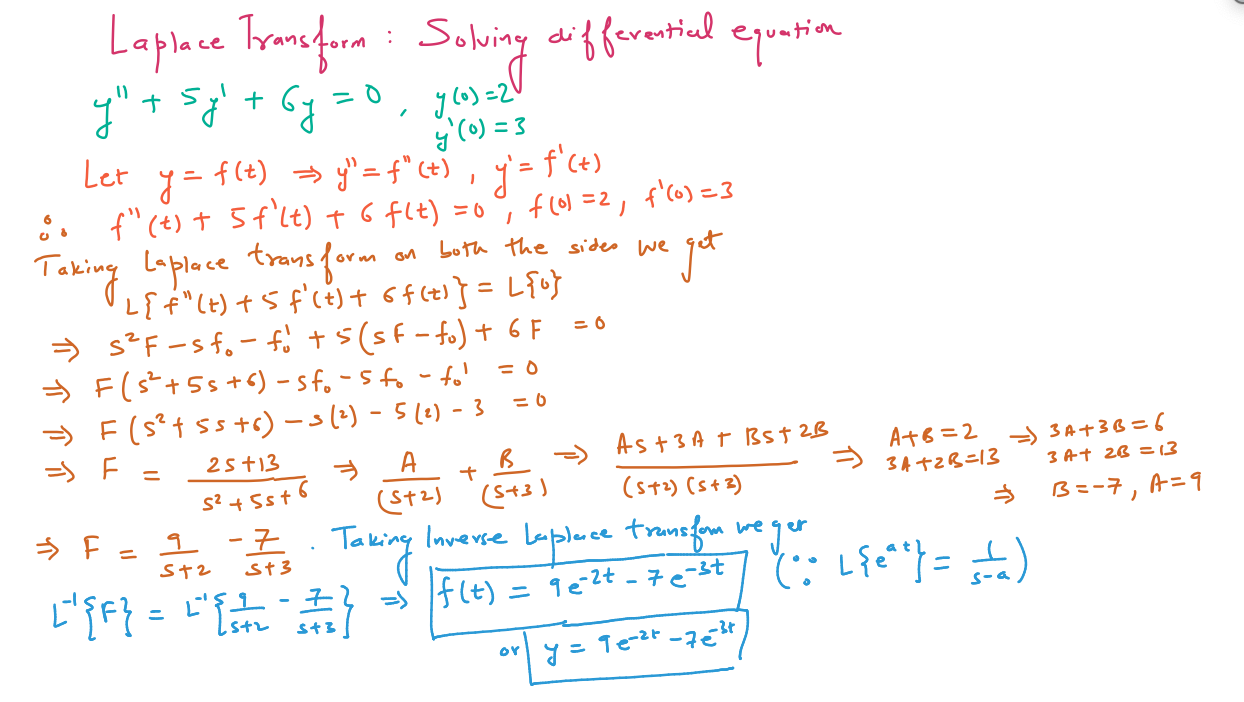
Introduction to Laplace Transforms

The Laplace transform is a powerful mathematical tool used to convert a function of time into a function of a complex variable. This transformation simplifies the process of solving linear ordinary differential equations, particularly when dealing with initial value problems. The Laplace transform is denoted as:

L{f(t)} = F(s) = ∫[0, ∞] e^(-st) f(t) dt

Where:

• f(t): The original function of time.
• F(s): The transformed function of the complex variable s.
• e^(-st): The exponential decay factor.
• dt: The infinitesimal time element.

Properties of Laplace Transforms

Understanding the properties of Laplace transforms is essential for effectively applying them in differential equations:

• Linearity: The Laplace transform is a linear operator, allowing for the transformation of sums and scalar multiples of functions.
• Time Shifting: The transform can handle time shifts in functions, making it useful for analyzing systems with delays.
• Frequency Shifting: This property allows for the adjustment of frequency components in the transformed function.
• Initial and Final Value Theorems: These theorems provide valuable insights into the behavior of the original function based on its Laplace transform.

Applications of Laplace Transforms

Laplace transforms find applications in various fields, including:

• Control Systems: Engineers use Laplace transforms to analyze and design control systems, ensuring stability and performance.
• Electrical Engineering: The transformation aids in solving circuit equations and analyzing transient responses.
• Mechanical Systems: Laplace transforms help model and solve differential equations related to mechanical vibrations and dynamics.
• Signal Processing: In signal processing, Laplace transforms are used to analyze and filter signals effectively.

Connection Between Differential Equations and Laplace Transforms

The connection between differential equations and Laplace transforms is vital for solving initial value problems. By applying the Laplace transform to a differential equation, we can transform it into an algebraic equation in the s-domain. This process simplifies the solution, allowing us to manipulate the equation more easily.

Once the algebraic equation is solved for F(s), the inverse Laplace transform is applied to revert back to the time domain, yielding the solution to the original differential equation. This method is particularly advantageous when dealing with complex systems and initial conditions.

Conclusion

In summary, understanding differential equations and Laplace transforms is crucial for anyone involved in mathematics, engineering, or the sciences. By mastering these concepts, individuals can effectively model and analyze dynamic systems, leading to better decision-making and problem-solving capabilities.

We encourage readers to explore further, practice solving differential equations, and apply Laplace transforms in various scenarios. If you have any questions or insights, feel free to leave a comment below or share this article with others who might benefit from it.

Thank you for reading! We hope this guide has enriched your understanding and inspired you to continue your learning journey in mathematics and engineering.