How To Find A Vertical Asymptote: A Comprehensive Guide

When it comes to understanding the behavior of functions, knowing how to find a vertical asymptote is crucial. Vertical asymptotes indicate where a function approaches infinity or negative infinity, signifying points where the function is undefined. This guide will walk you through the process of identifying vertical asymptotes, ensuring you grasp this fundamental concept in calculus and algebra.

In this article, we'll delve into the definition of vertical asymptotes, methods to find them, and important examples to illustrate each step. For students, educators, and math enthusiasts alike, mastering this topic is essential for tackling more complex mathematical concepts. By the end of this article, you will have a clear understanding of how to find vertical asymptotes in various functions.

Whether you're a high school student preparing for exams or someone looking to refresh your knowledge, this guide aims to provide a thorough exploration of vertical asymptotes. Let’s embark on this mathematical journey and uncover the intricacies of finding vertical asymptotes!

Table of Contents

• Definition of Vertical Asymptote
• How to Find Vertical Asymptotes
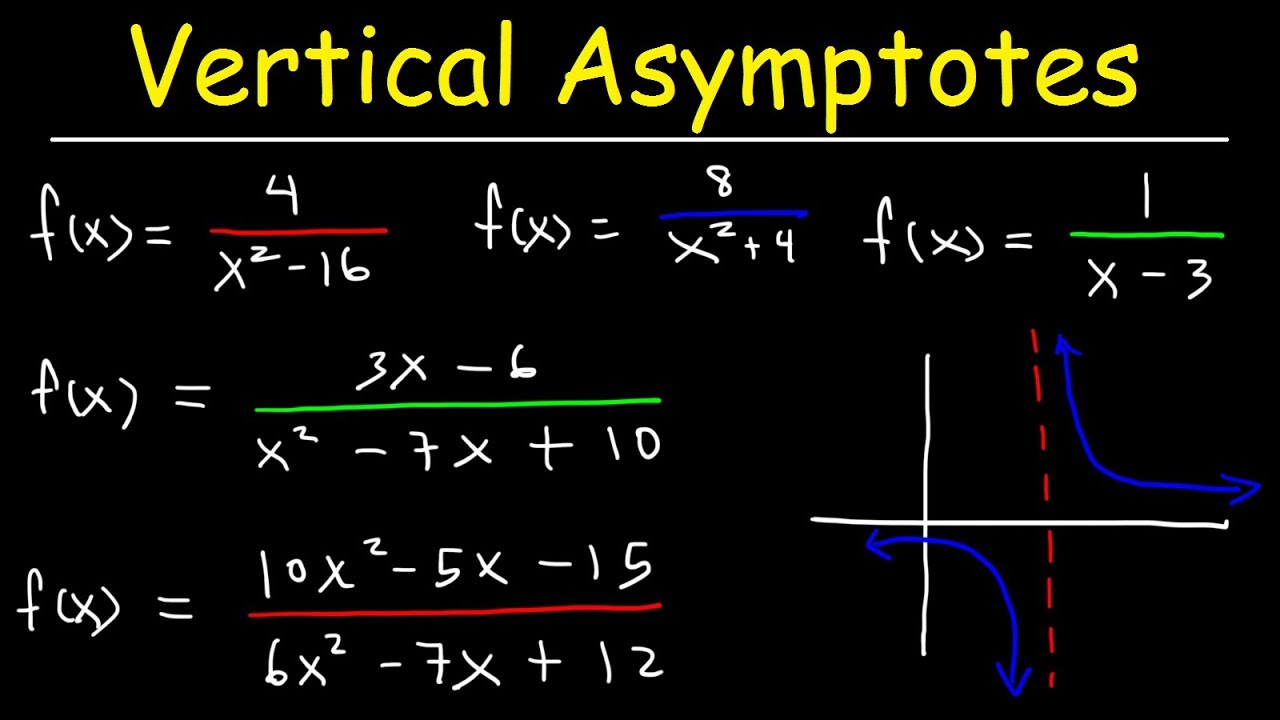
• Finding Vertical Asymptotes in Rational Functions
• Finding Vertical Asymptotes in Non-Rational Functions
• Examples of Vertical Asymptotes
• Graphical Analysis of Vertical Asymptotes
• Common Mistakes When Finding Vertical Asymptotes
• Importance of Vertical Asymptotes
• Conclusion

Definition of Vertical Asymptote

A vertical asymptote is a line that a graph approaches but never touches or crosses. It signifies that as the input values (x-values) approach a certain number, the output values (y-values) tend to either positive or negative infinity. Mathematically, this can be expressed as:

As x approaches a value 'a', f(x) approaches ±∞.

Vertical asymptotes often occur in rational functions, where the denominator equals zero, leading to undefined points. Understanding this concept is vital for analyzing the overall behavior of functions.

How to Find Vertical Asymptotes

Finding vertical asymptotes involves examining the function, particularly its denominator. Here are the steps to follow:

1. Identify the function and its domain.
2. Set the denominator equal to zero.
3. Solve for the values of 'x'.
4. Check if these values lead to a vertical asymptote by ensuring they do not cancel with the numerator.

Finding Vertical Asymptotes in Rational Functions

Rational functions are the most common type where vertical asymptotes are found. A rational function is defined as the ratio of two polynomials:

f(x) = P(x) / Q(x)

To find vertical asymptotes:

1. Set Q(x) = 0 and solve for x.
2. Check if these x-values cause the numerator P(x) to be non-zero.
3. If P(x) ≠ 0 at these points, they represent vertical asymptotes.

Finding Vertical Asymptotes in Non-Rational Functions

While vertical asymptotes are most commonly associated with rational functions, they can also occur in non-rational functions. For example, functions involving logarithms or certain trigonometric functions may have vertical asymptotes.

To find vertical asymptotes in these cases:

1. Identify the points where the function is undefined (such as log(x) where x ≤ 0).
2. Investigate the limits approaching these points.

Examples of Vertical Asymptotes

Let’s go through some examples to illustrate the process of finding vertical asymptotes:

Example 1: Rational Function

Consider the function:

f(x) = (x + 2) / (x^2 - 4)

To find the vertical asymptotes:

1. Set the denominator to zero: x² - 4 = 0.
2. Solve: x² = 4 → x = ±2.
3. Check the numerator: (x + 2) is non-zero at x = 2 (it equals 4) and zero at x = -2 (asymptote at +2).

Thus, there is a vertical asymptote at x = 2.

Example 2: Logarithmic Function

For the function:

g(x) = log(x - 1)

To find vertical asymptotes:

1. Identify where the function is undefined: x - 1 ≤ 0 → x < 1.
2. Check the limit: as x approaches 1 from the right, g(x) approaches -∞.

Therefore, there is a vertical asymptote at x = 1.

Graphical Analysis of Vertical Asymptotes

Graphing functions can provide visual insights into vertical asymptotes. Here are key points to consider:

• Vertical asymptotes appear as dashed lines on graphs.
• As the graph approaches the asymptote, the function values rise or fall dramatically.
• Check the behavior of the function on both sides of the asymptote to understand its limits.

Common Mistakes When Finding Vertical Asymptotes

While finding vertical asymptotes, students often make several common mistakes:

• Forgetting to check if the numerator cancels with the denominator.
• Not considering limits when dealing with non-rational functions.
• Assuming that all undefined points are vertical asymptotes.

Importance of Vertical Asymptotes

Understanding vertical asymptotes is essential for several reasons:

• They provide insight into the behavior of functions near critical points.
• Vertical asymptotes can indicate limits and continuity issues in calculus.
• Recognizing vertical asymptotes aids in sketching accurate graphs of functions.

Conclusion

In summary, finding vertical asymptotes is a fundamental skill in mathematics, particularly when dealing with rational and non-rational functions. By setting the denominator to zero and analyzing the behavior of the function, you can identify these critical points effectively. Remember to check for cancelation with the numerator to ensure accuracy.

Now that you have a comprehensive understanding of how to find vertical asymptotes, we encourage you to practice with various functions. Leave your comments below if you have any questions or insights, and don't forget to share this article with fellow students or anyone interested in mastering vertical asymptotes!

Thank you for reading, and we hope to see you again for more insightful mathematical discussions!